Department of Mathematics
Functional Analysis and Mathematical Physics Interdepartmental Research Group (FAMP)
Upcoming Colloquia
Date and Time: Friday, April 29, 2022, at 10:00 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Gabrile Martinez Lazaro (California State University, Fresno)
Title: On Linear Chaos in the Space of Convergent Sequences
Abstract: 
Recent Colloquia
Date and Time: Friday, February 25, 2022, at 11:00 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Christoph Fischbacher (University of California, Irvine)
Title: Area Laws for the Entanglement Entropy in the XXZ Spin Chain
Abstract: In this talk, we give a physical motivation why so-called Area Laws for the Entanglement Entropy are considered as indicators of Many-Body Localization (MBL). As it turns out, the difference between localization and delocalization seems to lie within a subtle logarithmic term. I will then present my results on the Entanglement Entropy for the Heisenberg XXZ Model, which is the first interacting many-particle model, for which MBL phenomena have been rigorously shown.
Date and Time: Friday, February 11, 2022, at 10:00 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Dr. Ahmed Farag Ali (University of California, Merced)
Title: Discreteness of Space and Phenomenological Implications
Abstract: Several approaches to quantum gravity suggest a quantum picture of spacetime. In this talk, we introduce a model to discretize the space through the modification of the uncertainty principle. We verify our model with the Schrödinger equation, Klein Gordon equation, and Dirac equation in both cases of few-body systems and field systems. We discuss the implications through tabletop experiments.
Date and Time: Friday, November 12, 2021, at 10:00 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Joey Contreras (Research Advisors: Drs. Douglas Singleton and Michael Bishop, Departments of Physics and Mathematics, California State University, Fresno)
Title: Modified Commutators vs. Modified Operators in a Quantum Gravity Minimal Length Scale
Abstract: Generic theories of quantum gravity often postulate that at some high energy/momentum scale there will be a fixed, minimal length. Such a minimal length can be phenomenologically investigated by modifying the standard Heisenberg Uncertainty relationship. This is often done by modifying the position-momentum commutator, which in turn means modifying the position and momentum operators. However, modifications which change the uncertainty relationships lead to conflicts with observational data (e.g., gamma ray bursts). These observations seem to imply that there is no minimal length scale. Meanwhile, modifying the operators, such that the standard uncertainty relation retains the same form, leads to no such conflict with observational data. We show that it is this modification of the position and momentum operators that is the key determining factor in the existence (or not) of a minimal length scale.
Date and Time: Friday, October 29, 2021, at 10:00 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Douglas Singleton, Ph.D. (California State University, Fresno)
Title: What is Spin?
Abstract: All electrons have the following properties: charge, mass and spin. Spin is an intrinsic
angular momentum but in almost all standard quantum mechanics texts the student is
warned that spin cannot be described as the rotating of anything; that spin is purely
a quantum property of the electron that cannot be thought of in terms of any classical
rotation. In this talk, we follow the arguments of an American Journal of Physics
article to show that this point of view is just wrong. We also discuss some other
interesting and unusual properties of the electron that arise due to its
spin.
Date and Time: Friday, October 15, 2021, at 11:00 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Michael Maroun , Ph.D. Development (Global Vice President of Research and TeXDyn Industries Corporate Laboratories Austin, TX)
Title: Singular Perturbations of Schrödinger Operators in Generalized Distributional Quantum Theory
Abstract: 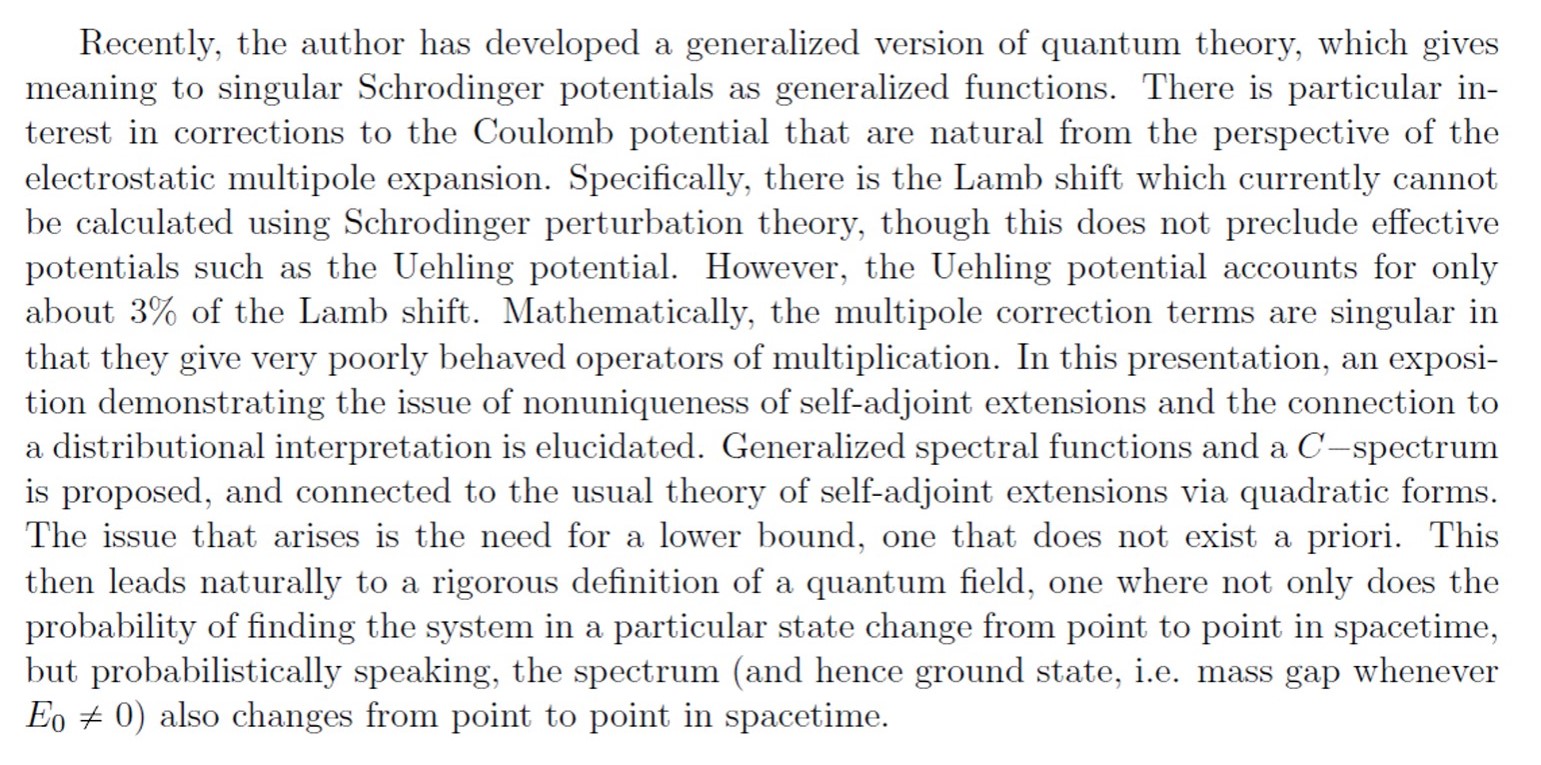
Date and Time: Friday, October 8, 2021, at 11:00 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Olivia Soghomonian, Student
Title: On a Characterization of Convergence in Banach Spaces with a Schauder Basis
Abstract: We extend the well-known characterizations of convergence in the spaces lp (1 \le p < \infty) of p-summable sequence and c0 of vanishing sequences to a general characterization of convergence in a Banach space with a Schauder basis and obtain as instant corollaries characterizations of convergence in an infinite-dimensional separable Hilbert space and the space of c convergent sequences.
The Functional Analysis, Mathematical Physics, and Dynamical Systems (FAMPDS) Joint American-Ukrainian Virtual Colloquium Series
Upcoming Colloquia
Date and Time: Friday, February 25, 2022, at 10:00 AM
Location: Via Zoom on the FAMP Zoom link.
Speaker: Oleksii Rebenko (Institute of Mathematics, National Academy of Sciences of Ukraine)
Title: The New simplest Proof of Ceyley's Formula and Its Connection to Statistical Mechanics
Abstract: A new very simple proof of the number of labeled rooted forest-graphs with a given number of vertices is furnished. As a particular case of this formula, we have Cayley's formula. A connection to cluster expansions of statistical mechanics is established.
Recent Colloquia
Date and Time: Friday, December 10, 2021, at 10 AM
Location: Zoom on the FAMP Zoom link
Speaker: Igor V. Nikolaev (St. John's University, New York City)
Title: Langlands Reciprocity for C*-Algebras
Abstract: 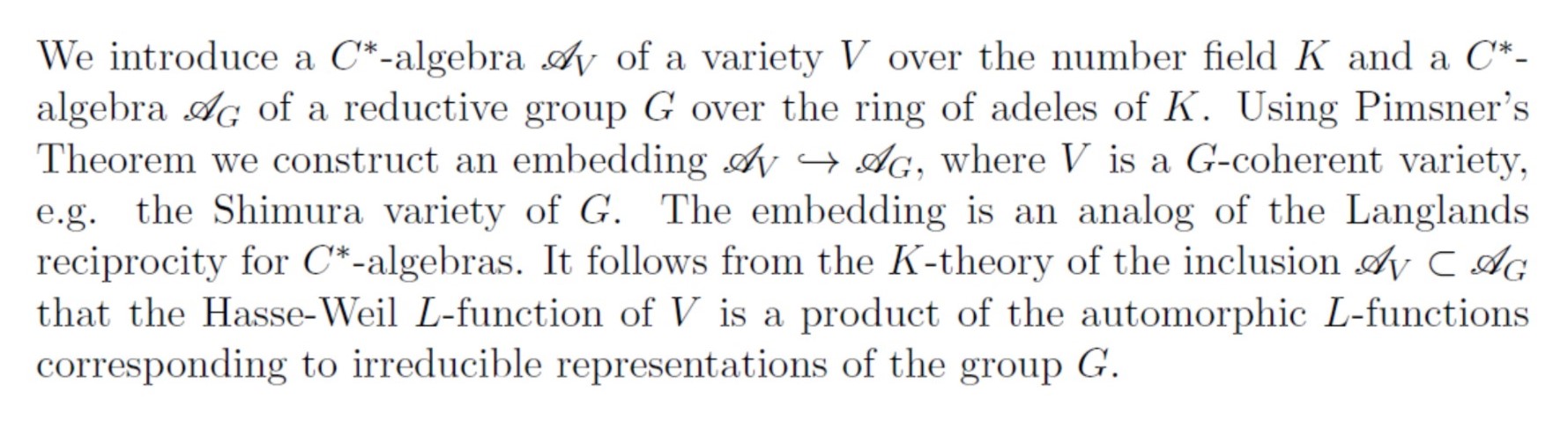
Date and Time: Friday, November 5, 2021, at 10 AM
Location: Zoom on the FAMP Zoom link
Speaker: Marat V. Markin (California State University, Fresno)
Title: On Spectral Inclusion and Mapping Theorems and Asymptotics for C0-Semigroups
Abstract: We establish spectral inclusion and mapping theorems for scalar type spectral operators, generalizing their counterparts for normal operators. Thereby, we extend a precise weak spectral mapping theorem along with the spectral bound equal growth bound condition and a generalized Lyapunov stability theorem, known to hold for C0-semigroups of normal operators on complex Hilbert spaces, to the more general case of C0-semigroups of scalar type spectral operators on complex Banach spaces. The finer spectrum structure is given itemized consideration. We also obtain exponential estimates with the best stability constants for such semigroups and extend to a Banach space setting a celebrated characterization of uniform exponential stability for C0-semigroups on complex Hilbert spaces, thereby acquiring a characterization of uniform exponential stability for scalar type spectral and eventually norm-continuous C0-semigroups.
Date and Time: Friday, October 22, 2021, at 10 AM
Location: Zoom on the FAMP Zoom link
Speaker: Yuri Latushkin (University of Missouri)
Title: Asymptotic Perturbation Theory for Extensions of Symmetric Operators
Abstract: In this talk (joint work with Selim Sukhtaiev (Auburn University)), we discuss asymptotic perturbation theory for varying self-adjoint extensions of symmetric operators. Employing symplectic formulation of self-adjointness we obtain a new version of Krein formula for resolvent difference, which facilitates asymptotic analysis of resolvent operators via first order expansion for the family of Lagrangian planes associated with perturbed operators. Specifically, we derive a Riccati-type differential equation and the first order asymptotic expansion for resolvents of self-adjoint extensions determined by smooth one-parameter families of Lagrangian planes. This asymptotic perturbation theory yields a symplectic version of the abstract Kato selection theorem and Hadamard-Rellich-type variational formula for slopes of multiple eigenvalue curves bifurcating from an eigenvalue of the unperturbed operator. The latter, in turn, gives a general infinitesimal version of the celebrated formula equating the spectral flow of a path of self-adjoint extensions and the Maslov index of the corresponding path of Lagrangian planes. Applications are given to quantum graphs, periodic Kronig-Penney model, elliptic second order partial differential operators with Robin boundary conditions, and physically relevant heat equations with thermal conductivity.
If you need a disability-related accommodation or wheelchair access information, please contact the mathematics department at 559.278.2992 or e-mail mathsa@csufresno.edu. Requests should be made at least one week in advance of the event.