Department of Mathematics
FAMP Colloquium - Fall 2020-Spring 2021
Date and Time: Friday, May 14, at 12 PM
Location: Via Zoom on the FAMP Zoom link
Speaker: Grygoriy Torbin (National Pedagogical Dragomanov University, Kyiv, Ukraine, Institute of Mathematics, National Academy of Sciences of Ukraine)
Title: On the Faithfulness of the Vitali Coverings for the Hausdorff Dimension Calculation and Its Applications
Abstract: One approach to the simplification of the calculation of the Hausdorff dimension consists in some restrictions of admissible coverings. In this talk, we discuss new results related to the faithfulness/nonfaithfulness of the Vitali coverings generated by different expansions for real numbers as well as some open questions in this direction. Another important topic to be treated is related to transformations preserving the Hausdorff dimension. We consider the interplay of this topic with fine fractal analysis of singularly continuous probability measures and faithfulness of the Vitali coverings. Finally, we discuss applications of the above results in dimensional number theory (fractal properties of subsets of non-normal numbers).
Date and Time: Friday, May 7, at 12 PM
Location: Via Zoom on the FAMP Zoom link
Speaker: Saurya Das (University of Lethbridge, Canada)
Title: Quantum Raychaudhuri Equation: Implications for Spacetime Singularities and the Quantum Origin of Lambda
Abstract: The Raychaudhuri equation predicts the convergence of geodesics and gives rise to the singularity theorems. The quantum Raychaudhuri equation (QRE), on the other hand, shows that quantal trajectories, the quantum equivalent of the geodesics, do not converge and are not associated with any singularity theorems. Furthermore, the QRE gives rise to the quantum corrected Friedmann equation. The quantum correction is dependent on the wavefunction of the perfect fluid whose pressure and density enter the Friedmann equation. We show that for a suitable choice of the wave-function this term can be interpreted as a small positive cosmological constant.
Date and Time: Friday, April 30, at 12 PM
Location: Via Zoom on the FAMP Zoom link
Speaker: Michael Dunia (Graduate Student, CSU, Fresno)
Title: Massive Photon, Magnetic Charge, and the Dirac Quantization Condition
Abstract: In this talk, we correct previous work on magnetic charge plus a photon mass. We argue that contrary to previous claims this system has a very simple, closed-form solution which is the Dirac string potential multiplied by an exponential decaying part. We present interesting features of this solution, namely: (i) the Dirac string becomes a real feature of the solution; (ii) the breaking of gauge symmetry via the photon mass leads to a breaking of the rotational symmetry of the monopole's magnetic field; (iii) the potential alteration of the Dirac quantization condition.
Date and Time: Friday, April 23, at 12 PM
Location: Via Zoom on the FAMP Zoom link
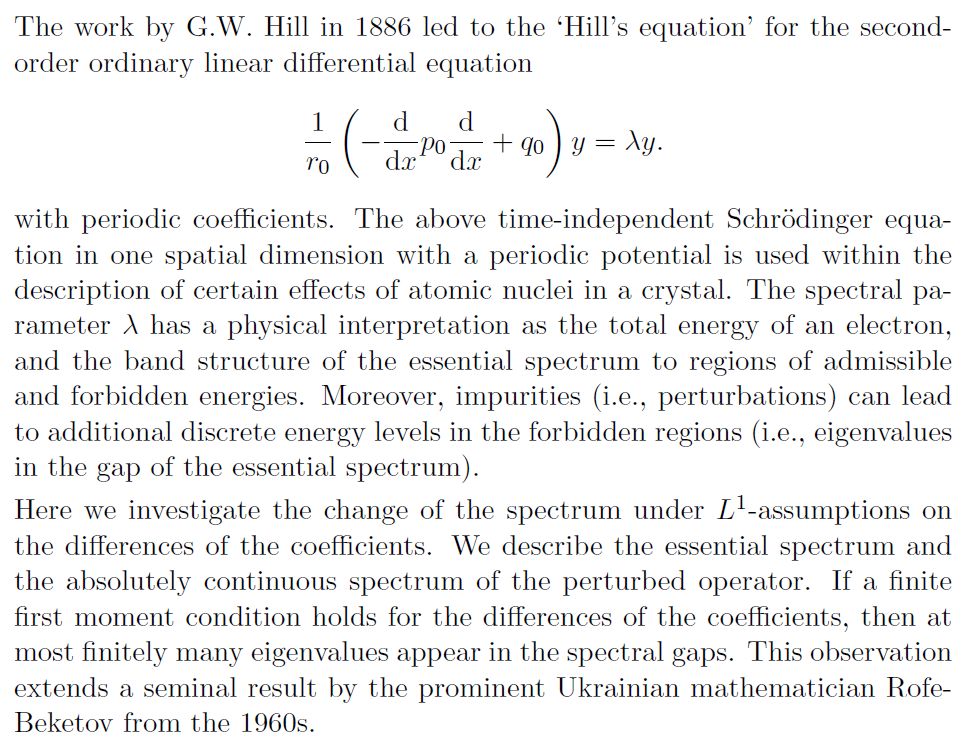
Speaker: Carsten Trunk (TU Ilmenau, Germany)
Title: Perturbations of Periodic Sturm-Liouville Operators
Abstract:
Date and Time: Friday, April 16, at 12 PM
Location: Via Zoom on the FAMP Zoom link
Speaker: Pasquale Bosso (University of Lethbridge, Canada)
Title: Quantum Gravity Phenomenology from the Generalized Uncertainty Principle
Abstract: One of the cornerstones of Quantum Mechanics (QM), Heisenberg's Uncertainty Principle (HUP), establishes that it is not possible to simultaneously measure with arbitrary precision both the position and the momentum of a quantum system. This principle, however, does not prevent one from measuring with infinite precision the system’s position. However, theories of Quantum Gravity, aiming to bridge between General Relativity and QM, predict the existence of a minimal observable length - a minimal uncertainty on the position generally of the order of the Planck length. This prediction results therefore in a contradiction with HUP, requiring a modification of the principle. This need gave rise to the Generalized Uncertainty Principle (GUP). In this talk, we show how the GUP can change known aspects of standard QM, leading to ways to test Quantum Gravity.
Date and Time: Friday, April 9, at 11 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Jean Renault (Institut Denis Poisson Université d'Orléans Rue de Chartres, France)
Title: The C*-Algebra of a Twisted Groupoid Extension
Abstract: A strong motivation for the study of C*-algebras is the theory of unitary representations of locally compact groups. In the seventies, M. Rieffel and P. Green developed respectively the theory of induced representations and Mackey normal subgroup analysis in a C*-algebraic framework. We discuss how Mackey's virtual groups program also fits into this framework and illustrate it by the example of projective representations of locally compact abelian groups.
Date and Time: Friday, March 26, at 12 PM
Location: Via Zoom on the FAMP Zoom link
Speaker: Marat V. Markin (California State University, Fresno)
Title: On Spectral Mapping Theorems and Asymptotics of Scalar Type Spectral C0-Semigroups
Abstract: We establish spectral inclusion and mapping theorems for scalar type spectral operators and thereby extend a weak spectral mapping theorem and a generalized Lyapunov stability theorem, known to hold for the C0-semigroups of normal operators on complex Hilbert spaces, to the more general case of the C0-semigroups of scalar type spectral operators on complex Banach spaces. For such semigroups, we also obtain a spectral mapping theorem for point spectrum, exponential estimates, and an analogue of the Gearhart-Prüss-Greiner characterization of the uniform exponential stability for C0-semigroups on complex Hilbert spaces.
Date and Time: Friday, March 19, at 12 PM
Location: Via Zoom on the FAMP Zoom link
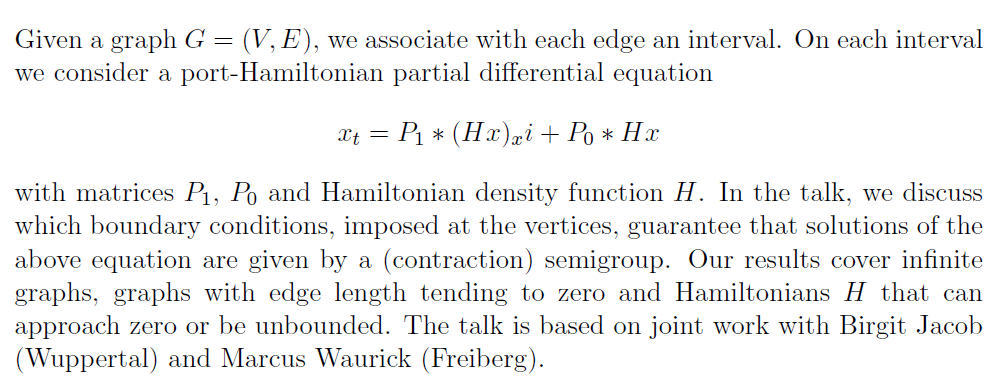
Speaker: Sven-Ake Wegner (University of Hamburg, Germany)
Title: Port-Hamiltonian Differential Equations on Infinite Networks
Abstract:
Date and Time: Friday, March 12, at 12 PM
Location: Via Zoom on the FAMP Zoom link
Speaker: Edward Sichel (FAMP, CSU Fresno)
Title: On the Non-Hypercyclicity of Normal Operators, Their Exponentials, and Symmetric Operators
Abstract:

Part of the Functional Analysis, Mathematical Physics, and Dynamical Systems (FAMPDS) Joint American-Ukrainian Virtual Colloquium Series
Date and Time: Friday, June 11, at 10 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Alexandra Antoniouk (Institute of Mathematics, National Academy of Sciences of Ukraine)
Title: Statistical Mechanics, Operator Semigroup Theory and the Problem of Differentiability in Initial Data for Nonlinear Equations
Abstract: Frequently, the development of mathematics is associated with problems emerging in
physics,
which pose new questions and require a fresh viewpoint at traditional matter. In this
talk, we consider an important class of models of statistical physics, the infinite
system of particles describing an anharmonic crystal, which furnishes a typical example
of an infinite-dimensional nonlinear dynamical system. The infinite dimensionality
of the system along with the nonlinearity of the model pose a two-fold complexity
to overcome. The natural question on the regular behavior of such a dynamical system
gives rise to the problem of the regularity of an operator semigroup, which need not
be strongly continuous. The question itself is related to the differentiability with
respect to initial data for operator Cauchy problems with unbounded nonlinear operators,
which appears to be yet unsolved in its full generality.
Date and Time: Friday, May 28, at 10 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Victor Feruk and Oleksandr Pokutnyi (Institute of Mathematics, National Academy of Sciences of Ukraine)
Title: Minimizing the Quadratic Functional on the Hopfield Networks
Abstract: We consider the continuous Hopfield model with a weak interaction of network neurons described by a system of differential equations with linear boundary conditions, and discuss the questions of finding necessary and sufficient conditions of solvability and construction of solutions of the given problem, turning into solutions of the linear generating problem, as the parameter ε tends to zero. We further construct an iterative algorithm for finding solutions with a quadratic rate of convergence and consider the problem of finding the extremum of the target functions on the problem’s solutions. The results are illustrated with examples for the case of three neurons.
Date and Time: Friday, May 21, at 10 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Viacheslav Rabanovich (Institute of Mathematics, National Academy of Sciences of Ukraine)
Title: On Decompositions of an Operator into a Sum of Projections
Abstract: Linear combinations of orthoprojections (in short, projections), in particular their sums, appear in various problems of operator theory and its applications. A classical result is the spectral theorem on decomposition of a self-adjoint operator with a finite spectrum into a linear combination of projections onto the eigenspaces, the projections being pairwise orthogonal. Excluding the orthogonality condition for projections leads to interesting. For instance, every bounded self-adjoint operator is a linear combination of 4 projections (or an integral combination of 5 projections). Also, operators from a wide class can be decomposed into sums of a small number of projections. Such decompositions are used in different numerical problems, especially where the calculation process can be parallelized, in information theory (frames as codes), and in quantum information theory. We discuss the known facts on sums of projections, describe general methods of constructing various decompositions, and partially consider numerical applications.
Date and Time: Friday, May 14, at 10 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Jean Renault (Institut Denis Poisson Université d'Orléans Rue de Chartres, France)
Title: The C*-Algebra of a Twisted Groupoid Extension
Abstract: A strong motivation for the study of C*-algebras is the theory of unitary representations of locally compact groups. In the seventies, M. Rieffel and P. Green developed respectively the theory of induced representations and Mackey normal subgroupvanalysis in a C*-algebraic framework. We discuss how Mackey's virtual groups program also fits into this framework and illustrate it by the example of projective representations of locally compact abelian groups.
Date and Time: Friday, May 7, at 10 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Michael Gekhtman (University of Notre Dame)
Title: Generalized Cluster Structures and Periodic Difference Operators
Abstract: We will consider a construction that ties together of several diverse notions including
spaces of periodic difference operators, Poisson sub manifolds of a Drinfeld double
of GL(n) and subsets of Grassmannians stable under the action of powers of a cyclic
shift. The theory of generalized cluster algebras serves as a unifying theme. Time
permitting, we will also discuss potential applications to representation theory
of quantum affine algebras at roots of unity.
Based on a joint work with M. Shapiro and A. Vainshtein and an ongoing project with C. Fraser and K. Trampel.
Date and Time: Friday, April 30, at 10 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Michel L. Lapidus (UC, Riverside)
Title: Can One Hear the Shape of a Fractal Drum?
Abstract: A well-known problem in mathematics and physics consists in understanding how the geometry (or shape) of a musical instrument affects it sound. This gives rise to two related types of mathematical problems: direct spectral problems (how the shape of a drum affects its sound) and inverse spectral problems (how one can recover the shape of a drum from its sound). Here, we consider both types of problems in the context of drums with fractal (that is, very rough) boundary. We show, in particular, that one can “hear” the fractal dimension of the boundary (a certain measure of its roughness) and, in certain cases, a fractal analog of its length. In the special case of vibrating fractal strings (the one-dimensional situation), we show that the corresponding inverse spectral problem is intimately connected with the Riemann Hypothesis, which is arguably the most famous open problem in mathematics and whose solution will likely unlock deep secrets about the prime numbers. In conclusion, we briefly explain how this work eventually gave rise to a mathematical theory of complex fractal dimensions (developed by the author and his collaborators), which captures the vibrations that are intrinsic to both fractal geometries and the prime numbers.
Date and Time: Friday, April 23, at 10 AM
Location: Via Zoom on the FAMP Zoom link
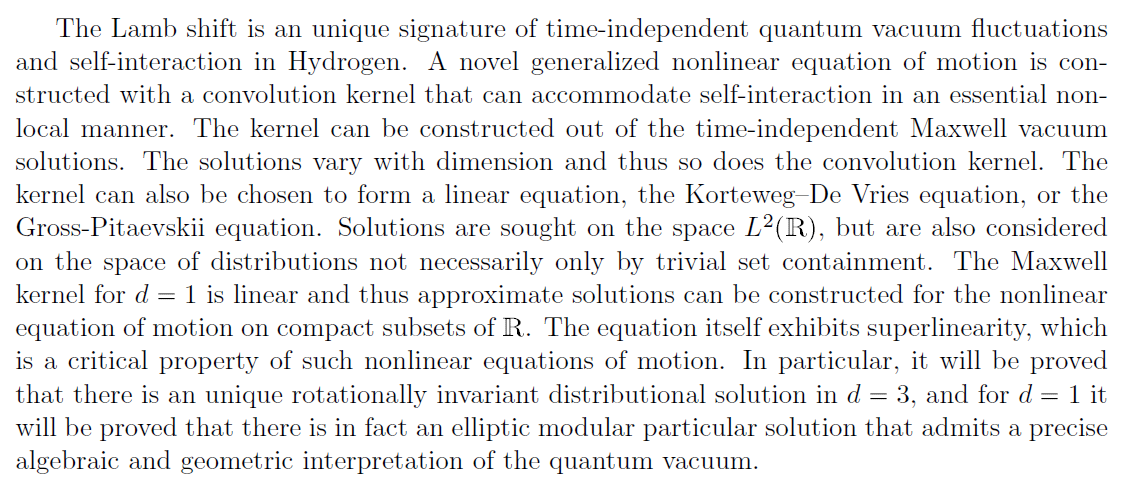
Speaker: Michael Maroun (Independent Researcher, Boston, MA)
Title: Generalized Nonlinear Schrödinger Equation: Self-Dynamics by Convolution
Abstract:
Date and Time: Friday, April 16, at 10 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Oleksandr Baranovskyi (Institute of Mathematics, National Academy of Sciences of Ukraine)
Title: Fractal Analysis of Singular, Nowhere Differentiable, and Nowhere Monotonic Functions
Abstract: We give an overview of research topics at the Department of Dynamical Systems and Fractal Analysis. In particular, various systems of encoding (representation) for real numbers with finite and infinite alphabet are discussed. We use these systems for analytical definition and studying some mathematical objects with complicated local structure. Singular probability distribution functions, nowhere differentiable functions, and nowhere monotonic functions are among them. We study fractal properties of such functions, i.e., fractal properties of their level sets, graphs, and other related sets.
Date and Time: Friday, March 26, at 9 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Vesselin G. Gueorguiev (Institute for Advanced Physical Studies, Bulgaria, Ronin Institute for Independent Scholarship, USA)
Title: Revisiting the Cosmological Constant Problem within Quantum Cosmology
Abstract: A new perspective on the Cosmological Constant Problem (CCP) is proposed and discussed within the multiverse approach of Quantum Cosmology. It is assumed that each member of the ensemble of universes has a characteristic scale a that can be used as integration variable in the partition function. An averaged characteristic scale of the ensemble is estimated by using only members that satisfy the Einstein field equations. The averaged characteristic scale is compatible with the Planck length when considering an ensemble of solutions to the Einstein field equations with an effective cosmological constant. The multiverse ensemble is split in Planck-seed universes with vacuum energy density of order one; thus, Λ̃≈8π in Planck units and a-derivable universes. For a-derivable universe with a characteristic scale of the order of the observed Universe a≈8×1060, the cosmological constant Λ=Λ̃/a2 is in the range 10−121 − 10−122, which is close in magnitude to the observed value 10−123. We point out that the smallness of Λ can be viewed to be natural if its value is associated with the entropy of the Universe. This approach to the CCP reconciles the Planck-scale huge vacuum energy-density predicted by QFT considerations, as valid for Planck-seed universes, with the observed small value of the cosmological constant as relevant to an a-derivable universe as observed.
Date and Time: Friday, March 12, at 10 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Selden Crary (Independent Researcher, Palo Alto, California, USA)
Title: The Algebra and Geometry of Twin-Point Designs in Optimal Design of Computer Experiments
Abstract: We continue our introduction to optimal clustered designs in statistical design of computer experiments. (1) New experimental evidence: The emergence of a hierarchy of clustered designs, such as pairs of twin-point designs. (2) New theory: A theorem stating the integrated-mean-squared-prediction-error objective function is a low-degree-truncated, rational, generalized function; the connection of this “Nuclass” of function with total positivity and of total positivity with the Riemann hypothesis; and a theorem stating that the assumption of invariance of the algebra of twins, over their design domain, leads to curvature of the domain. (3) Speculated applications, beyond design of experiments: Superconductivity, topologically protected states of matter, biological regeneration, and anti-senescence.
Date and Time: Friday, March 19, at 10 AM
Location: Via Zoom on the FAMP Zoom link
Speaker: Volodymyr Koshmanenko (Institute of Mathematics, National Academy of Sciences of Ukraine, Interdisciplinary Research Center for Complex Systems, National Pedagogical University, Kyiv)
Title: Setting of the Conflict Problem in the Framework of Functional Analysis
Abstract: We give an introduction to the mathematical setting of the conflict problem in terms of constructions in a Hilbert space. The opponents, as the main objects of conflict phenomena, will be presented by operators and vectors in a Hilbert space or by probability distributions on a joint resource space for opponents. The conflict phenomenon is mathematically reflected as the intersection or overlap of operator domains and spectral measure supports. The confrontation between opponents is described by the conflict composition (a transformation in terms of spectral measures). It generates the conflict dynamical system. Thus, there emerges the fundamental question of how to redistribute the starting intersections and overlaps.
The resolution of a conflict problem means arriving at equilibrium states (fixed points) for the conflict dynamical system.
Date and Time: Friday, March 12, at 10 AM
Location: Via Zoom on the FAMP Zoom link
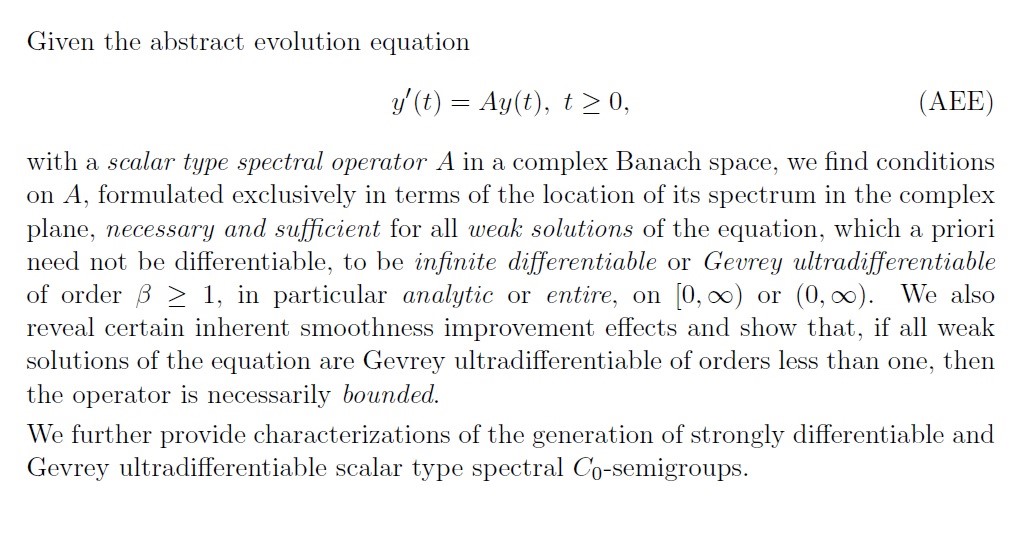
Speaker: Marat V. Markin (California State University, Fresno)
Title: On the Smoothness of Weak Solutions of an Abstract Evolution Equation with a Scalar Type Spectral Operator
Abstract:
Date and Time: Friday, February 26, 2021, at 10:00 AM
Location: Zoom at Zoom Link for FAMP
Speaker: Dr. Anatoly N. Kochubei (Institute of Mathematics, National Academy of Sciences of Ukraine)
Title: Non-Archimedean Radial Calculus
Abstract:
Functional Analysis, Mathematical Physics, and Dynamical Systems (FAMPDS) Winter 2021 Virtual Workshop
The event, featuring four talks, will take place on March 5, 2021, from 10 AM-3 PM.
The workshop is intended to create and consolidate contacts and foster collaborative opportunities between the scholars and graduate students involved in research in functional analysis, mathematical physics, dynamical systems, fractal, arithmetic, and noncommutative geometry, number theory, as well as other areas of interest. The talks are to be kept at the level accessible to the graduate students and non-experts.
Date and Time: Friday, February 26, 2021, at 12:00 PM
Location: Zoom at Zoom Link for FAMP
Speaker: Michael Maroun, Ph.D. (Independent Researcher, Boston, MA)
Title: Exact Solutions of the Local Linearization Associated with the One-Dimensional Nonlinear Schrödinger Equation
Abstract:
Date and Time: Friday, February 19, 2021 at 11:00 AM
Location: Zoom at Zoom Link for FAMP
Title: Perfectoid Modular Curves and Langlands Correspondence
Speaker: Shanna Dobson (California State University, Los Angels)
Abstract:
Date and Time: Friday, December 11, 2020 at 11:00 AM
Location: Zoom at Zoom Link for FAMP
Title: From Rainbows to Resurgence: Asymptotics of the Airy Function
Speaker: Will Hoffer (University of California, Riverside)
Abstract: In this talk, we take a modern perspective on the problem of finding the Stokes behavior of the Airy function through Borel resummation of its asymptotic expansion. In particular, we find that an ordinary asymptotic power expansion (when the parameter approaches infinity along the positive real axis) is missing exponentially small terms. Notably, these exponential terms become dominant as the phase of the parameter changes, and this switching on is directly responsible for the Stokes phenomenon. The primary result, then, is that the full analytic behavior of the Airy function resurges from the original expansion on the positive real line. This perspective can be thought of as resurgence analysis on a perturbative approach to the problem.
Date and Time: Friday, December 4, 2020 at 11:00 AM
Location: Zoom at Zoom Link for FAMP
Title: The Energy Eigenvalue for the Singular Wave Function of the Three Dimensional Dirac Delta Schrödinger Potential via Distributionally Generalized Quantum Mechanics
Speaker: Dr. Michael Maroun (Independent Researcher, Boston, MA)
Abstract: Unlike the situation for the 1d Dirac delta derivative Schrödinger pseudo potential (SPP) and the 2d Dirac delta SPP, where the indeterminacy originates from a lack of scale in the first and both a lack of scale as well as the wave function not being well defined at the support of the generalized function SPP; the obstruction in 3d Euclidean space for the Schrödinger equation with the Dirac delta as a SPP only comes from the wave function (the 𝐿 2 bound sate solution) being singular at the compact point support of the Dirac delta function (measure). The problem is solved here in a completely mathematically rigorous manner with no recourse to renormalization nor regularization. The method involves a distributionally generalized version of the Schrödinger theory as developed by the author, which regards the formal symbol “𝐻𝜓” as an element of the space of distributions, the topological dual vector space to the space of smooth functions with compact support. Two main facts come to light. The first is the bound state energy of such a system can be calculated in a well-posed context, the value of which agrees with both the mathematical and theoretical physics literature. The second is that there is then a rigorous distributional version of the Hellmann-Feynman theorem.
Date and Time: Friday, November 20, 2020 at 2:00 PM
Location: Zoom at Zoom Link for FAMP
Title: An Introduction to Complex Dimensions: The Case of Fractal Strings
Speaker: Michel L. Lapidus, Distinguished Professor of Mathematics, Burton Jones Endowed Chair in Pure Mathematics (Department of Mathematics, University of California, Riverside)
Abstract: We provide an introduction to the mathematical theory of complex fractal dimensions (developed by the author and his collaborators), which captures the vibrations that are intrinsic to both fractal geometries and the prime numbers. We focus here on the case of fractal strings, or one-dimensional drums with fractal boundaries. Complex dimensions are the poles of suitably defined geometric zeta functions associated to fractal strings. Intuitively, their real and imaginary parts correspond respectively to the amplitudes and the frequencies of “geometric waves” traveling through the space of scales associated with the fractal string. Explicit formulas, significantly extending Riemann’s original explicit formula for the prime number counting functional and the Riemann zeros, enable us to express very precisely the oscillations intrinsic to fractal and arithmetic geometries, via the underlying complex dimensions. Key examples of such formulas are fractal tube formulas and spectral asymptotic formulas with complex exponents, along with formulas for the prime orbit counting functions of certain dynamical systems generalizing the dynamical counterpart of the Prime Number Theorem. We will illustrate aspects of the theory by means of the Cantor string as well as via self-similar strings, the complex dimensions of which happen to exhibit generically very intriguing quasiperiodic patterns, which we conjecture to form (generalized) quasicrystals. In the next lecture, we plan to discuss the higher-dimensional theory of complex dimensions, and of the corresponding fractal zeta functions. The main reference for this talk is M. L. Lapidus and M. van Frankenhuijsen, Fractal Geometry, Complex Dimensions and Zeta Functions, Springer Monographs in Mathematics, Springer, New York, 2013 (second revised and enlarged edition).
Date and Time: Friday, November 13, 2020 at 11:00 AM
Location: Zoom at Zoom Link for FAMP
Title: In How Many Dimensions Do We Live?
Speaker: Dr. Piero Nicolini (Frankfurt Institute for Advanced Studies, Institute for Theoretical Physics, Goethe University Frankfurt, Germany)
Abstract: In this talk, we introduce the concept of dimension. We are to see that dimensions depend on the process of measurement and on the dynamics of a physical system. Then, we analyze the reasons that support the existence of a higher dimensional Universe. Finally, we discuss the alternative scenario of dimensionally reduced spacetimes.
Date and Time: Friday, November 6, 2020 at 11:00 AM
Location: Zoom at Zoom Link for FAMP
Title: Asymptotic Analysis of the Boltzmann Equation for Dark Matter Relic Abundance
Speaker: Jaryd Ulbricht (University of California, Santa Cruz)
Abstract: A solution to the Boltzmann equation governing the thermal relic abundance of cold dark matter is constructed by matched asymptotic approximations, using a uniform WKB method for large temperatures. The approximation of the relic density is an asymptotic series valid when the abundance does not deviate significantly from its equilibrium value until small temperatures. Resonance and threshold effects are taken into ac-count at leading order by approximating the thermally averaged cross section when the temperature is small compared to the mass of the dark matter particle. We compare our results to a numerical determination of the relic abundance using a benchmark model and find a fantastic agreement.
Date and Time: Friday, October 30, 2020 at 11:00 AM
Location: Zoom at Zoom Link for FAMP
Title: Spectral Bounds for Damped Systems
Speaker: Dr. Carsten Trunk (TU Ilmenau, Germany)
Abstract:
Date and Time: Friday, October 23, 2020 at 11:00 AM
Location: Zoom at Zoom Link for FAMP
Title: Metric Convergence of Spectral Triples on the Sierpinski Gasket and Other Piecewise C^1-fractal Curves
Speaker: Therese-Marie Landry (a Ph.D. Student, UC Riverside)
Abstract: Many important physical processes are often formulated in terms of operators on smooth manifolds. A natural question is to determine whether differential structures defined on fractals can be realized as a metric limit of differential structures on their approximating finite graphs. One of the fundamental tools of noncommutative geometry is Alain Connes' spectral triple. Spectral triples generalize differential structure and open promising avenues for extending analytic methods from mathematical physics to fractal spaces. The Sierpinski gasket can be viewed as a piecewise C1-fractal curve, a class of fractals first formulated by Michel Lapidus and Jonathan Sarhad. In this talk, we motivate and describe how their framework was adapted to our setting to yield approximation sequences suitable for metric approximation of spectral triples on piecewise C^1-fractal curves.
Date and Time: Friday, October 16, 2020 at 2:00 PM
Location: Zoom at Zoom Link for FAMP
Title: K-Theory of Etesi C*-Algebras
Speaker: Igor Nikolaev, Ph.D. (St. John’s University)
Abstract: 
Date and Time: Friday, October 9, 2020 at 11:00 AM
Location: Zoom at Zoom Link for FAMP
Title: On weak spectral mapping theorems, spectral structure, and asymptotics of C0-semigroups generated by scalar type spectral operators
Speaker: Marat V. Markin (Department of Mathematics, CSU, Fresno)
Abstract: We prove a weak spectral mapping theorem for scalar type spectral operators and apply it to extend a weak spectral mapping theorem and the generalized Lyapunov stability theorem, known to hold for the C0-semigroups of normal operators on complex Hilbert spaces, to the more general case of C0-semigroups of scalar type spectral operators on complex Banach spaces. For such semigroups, we also reveal finer spectral structure, obtain exponential estimates, and establish an analogue of the Gearhart-Prüss-Greiner characterization of the uniform exponential stability for C0-semigroups on complex Hilbert spaces.
The new results are to be presented for the first time.
Date and Time: Friday, October 2, 2020 at 11:00 AM
Location: Zoom at Zoom Link for FAMP
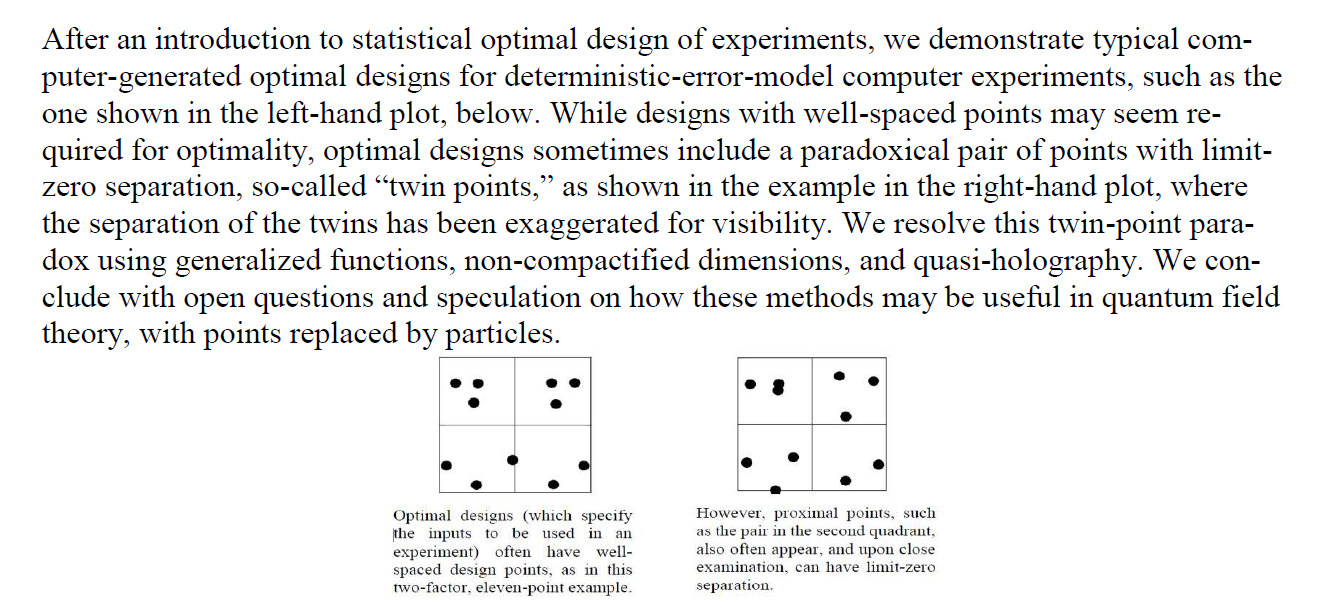
Title: The Twin-Point Paradox in the Design of Computer Experiments
Speaker: Selden Crary (Independent Researcher, Palo Alto, California, USA)
Abstract:
Date and Time: Friday, September 25, 2020 at 4:00 PM
Location: Zoom at Zoom Link for FAMP
Title: Gravitational Casimir Effect
Speaker: Dr. James Quach (University of Adelaide, Australia)
Abstract: The gravitational Casimir effect with non-idealized boundary conditions is derived. This allows the quantification of the gravitonic contribution to the Casimir effect from real bodies. We show how to calculate the meager gravitonic Casimir effect in ordinary matter. We also consider an application to the speculated Heisenberg-Coulomb (HC) effect in superconductors, thereby providing a test for the validity of the HC theory, and, consequently, the existence of gravitons.
Date and Time: Friday, September 18, 2020 at 10:50 AM
Location: Zoom at Zoom Link for FAMP
Title: Reconciling a Quantum Gravity Minimal Length with Lack of Dispersion
Speaker: Jaeyeong Lee (Department of Physics, CSU, Fresno)
Abstract: Generic arguments lead to the idea that quantum gravity has a minimal length scale. A possible observational signal of such a minimal length scale is that photons should exhibit dispersion. In 2009 the observation of a short gamma ray burst seemed to bind the minimal length scale to distances smaller than the Planck length, implying that space-time appeared continuous to distances below the Planck length. This poses a challenge for such minimal distance models. Here, we propose a modification of the position and momentum operators, x̂ and p̂, which lead to a minimal length scale, but preserve the photon energy-momentum relationship E = pc so that there is no dispersion of photons with different energies. This can be accomplished without modifying the commutation relation [x̂, p̂] = iħ.